Dr Omer Bobrowski

My main research area is random topology and its applications. This field is driven both by deep theoretical questions and the demands of state-of-the-art data analysis applications. This study combines theory and methods from various mathematical disciplines, including probability, statistics, combinatorics, topology, and geometry. My current research focuses on studying theoretical problems in random topology, notably, generalising questions on random graphs (e.g., connectivity, cyclicity, percolation, spectral properties) to higher-dimensional objects. Additionally, I work on applied problems bridging random topology and statistics. In recent years, the field of topological data analysis (TDA) has introduced a variety of versatile tools to extract structural information from highly generic data settings. These tools have been proven useful for many applications in diverse fields (e.g., cancer research, neuroscience, materials engineering, climate science, etc.). My research develops solid probabilistic foundations for TDA, which is essential for turning TDA methods into powerful statistical tools.

I have recently been awarded an EPSRC grant for the project titled “Topological Phase Transitions in Stochastic Geometry”. This project is on topological phenomena that exhibit abrupt changes. The goal is to analyse when and how such changes occur, and to extract insights from these phenomena that can be helpful for TDA.

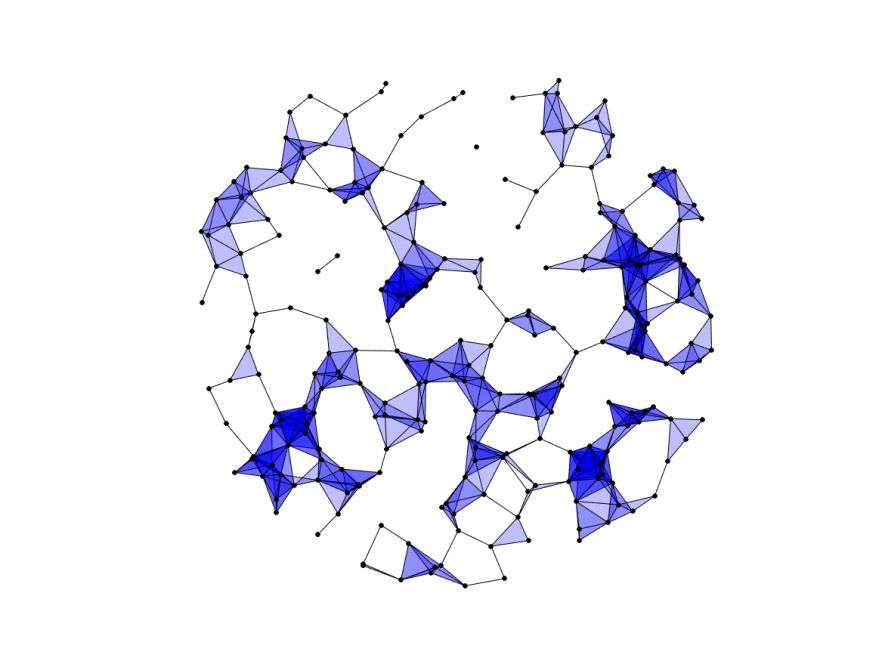
A common model to generate shape from random data points. This structure is called “a simplicial complex” and it is made of vertices, edges, triangles, tetrahedra, and higher dimensional objects (known as simplexes).